A. Pythagorean Theorem II
time limit per test
memory limit per test
input
output
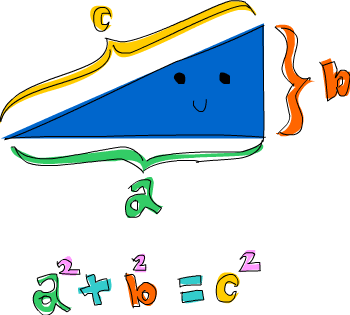
In mathematics, the Pythagorean theorem — is a relation in Euclidean geometry among the three sides of a right-angled triangle. In terms of areas, it states:
In any right-angled triangle, the area of the square whose side is the hypotenuse (the side opposite the right angle) is equal to the sum of the areas of the squares whose sides are the two legs (the two sides that meet at a right angle).
a, b and c, often called the Pythagorean equation:
a2 + b2 = c2
c represents the length of the hypotenuse, and a and b
n, your task is to count how many right-angled triangles with side-lengths a, b and c that satisfied an inequality 1 ≤ a ≤ b ≤ c ≤ n.
Input
n (1 ≤ n ≤ 104)
Output
Print a single integer — the answer to the problem.
Sample test(s)
input
5
output
1
input
74
output
35
显然n^2暴力枚举
注意要加优化-if (i*i+j*j>n*n) break. 不然我的n^2过不了
#include<cstdio>#include<cstring>
#include<cstdlib>
#include<algorithm>
#include<functional>
#include<iostream>
#include<cmath>
#include<cstring>
#include<cctype>
#include<ctime>
using namespace std;
#define For(i,n) for(int i=1;i<=n;i++)
#define Fork(i,k,n) for(int i=k;i<=n;i++)
#define Rep(i,n) for(int i=0;i<n;i++)
#define Forp(x) for(int p=pre[x];p;p=next[p])
int main()
{
// freopen(".in","r",stdin);
// freopen(".out","w",stdout);
int n;
cin>>n;
int ans=0;
For(i,n)
for(int j=i+1;j<=n;j++)
{
int c=i*i+j*j;
if (c>n*n) break;
double x=sqrt(c);
if (abs((x-(int)x))<1e-8) ans++;
}
cout<<ans<<endl;
return 0;
}
